The Computational Envelope of Language

Let me review. In Words, Binding, and Conversation as Computation I argued that a signifier has no meaning until it is bound to one in a linguistic act. The informal notion of a word as a sort of brief case in which the case itself is the signifier, the physical substance, while the contents is the meaning, or meanings, that notion is misleading at best. While linguists know this, I suspect that much linguistic thinking is still dominated by this notion and the professional corrections and modifications are like the cycles and epicycles needed to make geocentric astronomy work. Syd Lamb has a much better conception in Linguistic structure: A plausible theory (pp. 11 ff.).

In that post I went on to argue that conversation involves computation in the sense that I’m getting at, computation distributed over two individuals. The listener is binding the speaker’s lexemes (to use Lamb’s term) to (their own) meanings and the speaker is uttering lexemes that around bound to (their own internal) meanings. In the next post, What’s Computation? What’s Literary Computation?, I suggest that the brain is not computational through and through, but only in a certain aspect. Yes, it can all be simulated, but we can simulate nuclear reactions too; that doesn’t mean that nuclear reactions are computational. So it is only in some sense that the brain is computational. What sense? There’s “the binding of sound to sense, and there’s the mechanisms of conversational repair.”

That brings us to the third post in this series, Writing, computation and, well, computation, where I point out that if anything is computation, surely it is arithmetic. But arithmetic calculation arrived relatively late in human cultural evolution and is not ‘primitive’ or ‘natural’ to us. Moreover it is relatively difficult to do, requiring hours of explicit drill and practice over a half-dozen years or so.

What’s the basic computational activity in which arithmetic is based? What’s concentrated and distilled to make arithmetic, among other things?

I come back to binding lexemes to meanings, to sememes in Lamb’s terminology. That’s the primitive computational act.

Think of the young child just acquiring language. The child sees and interacts with the world around her. And of course interacts with her mother. Much of this interaction is purely physical and even talk is accompanied by gestures and postures of various kinds. In particular, we know from work by Paul Bloom (How Children Learn the Meanings of Words, MIT Press, 2000), that is not sufficient for the lexeme (as utterance) and its referent to be co-present to the child in order for the child to learn the association. The child must also observe her mother attending to the referent in some way. It is not simply that mother utters /doggie/ or /ball/, but that mother attends to them and the child see that.

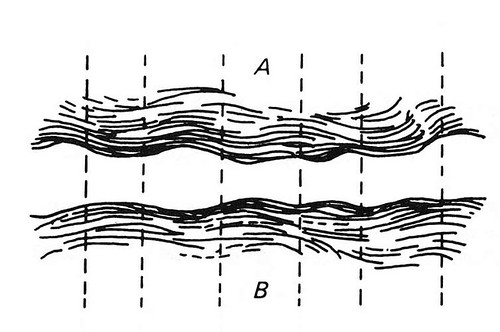
Making the association thus requires a relatively sophisticating perceptual and cognitive act. There’s much more going on than simply ‘attaching’ a signifier to a signified. Let us once again look at Saussure’s wave diagram [1]:

We’ve got the world of “jumbled ideas” at A and the continuous sounds of B. The child has got to segment both and bind sounds to sense. It is that double-act of segmentation (or digitization) and subsequent binding that I’m thinking is the basic, the atomic, act of computation. However that act operates ‘internally,’ however it is distributed over various functional areas of the brain, the ‘envelope’ of that act is a computation.

Initially the child does each such computation de novo, as it were, and it takes while to get the sound right (both perceptually and vocally) and get it linked to the right meaning. The child is learning many words and meanings more or less simultaneously and must make mutual adjustments across the lot of them – that’s what you do in a relational network. As time goes on the segmentations and the bindings become surer and more precise, less effortful, more routine. They come to seem automatic.

And so the linguistic sign (to use Saussure’s term) is born. The child now ‘has’ words, and it becomes increasingly easy to think of them as things, more so as the child learns to read and write. Even as the child acquires individual words, she also acquires syntactic patterns, linking words together. Those patterns extend the basic computational act, the binding of lexeme to sememe, across strings of lexemes and correlative collections of sememes.

THAT’s the computational envelope of language. And I think that’s enough to motivate my assertion that literary form is fundamentally computational. We’ll see.

* * * * *

[1] Ferdinand de Saussure, Course in General Linguistics, tr. Wade Baskin, ed. Perry Meisel and Haun Saussy, New York: Columbia University Press, 1987, 2011.